- Messages
- 1,171
- Reaction score
- 4,151
- Points
- 273
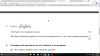
The weight, X grams, of soup put in a tin by machine A is normally distributed with a mean of 160 g and a standard deviation of 5 g. A tin is selected at random.
(a) Find the probability that this tin contains more than 168 g
Help plz
(a) Find the probability that this tin contains more than 168 g
Help plz