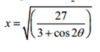Mathematics: Post your doubts here!

[areeba240]

Ouch forgot to tell you this is from the answer to part (ii), sorry about that. The solution to that DE is r = 25 / (5 - 2t).

If you are interested in how this function is obtained, here's my work for part (ii).
View attachment 61575

thank u

 

[Rahul Athreya]

a woman finds that she only gets home from work in time to see the start of the programme on average twice in a working week of five days, each

day being equally likely.

a) Find the probability that in a given working week she will be home in time to see the start of the programme:

iii) on 4 consecutive days but not the fifth.

b) Find the mean and standard deviation of the number of times she is home to see the start of the

programme during a period of six working weeks.

Thanks guys <3

 

[areeba240]

does anyone have cie nov. 2016 paper?

 

[Thought blocker]

does anyone have cie nov. 2016 paper?

http://papers.gceguide.com/A Levels/Mathematics (9709)/

 

[areeba240]

how to do 6(ii)

 

[DragonCub]

View attachment 61582
how to do 6(ii)

Though the focus is on (ii), it is based on part (i) which takes much more work.

I will put my work through part (i) as well. You can use it to check your answer.

 

[areeba240]

Though the focus is on (ii), it is based on part (i) which takes much more work.

I will put my work through part (i) as well. You can use it to check your answer.

View attachment 61583

Now onto part (ii):
View attachment 61585

the ans is wrong........

i got the equation givein in part (i) if possible plzz tell me how to find least value using this equation

 

[areeba240]

http://papers.gceguide.com/A Levels/Mathematics (9709)/9709_w16_qp_33.pdf

how to justify....???

how to do the 4(i)???

 

[DragonCub]

the ans is wrong........
View attachment 61586
i got the equation givein in part (i) if possible plzz tell me how to find least value using this equation View attachment 61587

Uh I'm soooo sorry, misread a tiny part of the question and that happened...

I've corrected my work (edited my original reply), and arrived at the same answer provided by the marking scheme.
Should not be any more errors there.

Again, sorry about the wrong answer

 

[DragonCub]

http://papers.gceguide.com/A Levels/Mathematics (9709)/9709_w16_qp_33.pdf
View attachment 61588
how to justify....???
View attachment 61589
how to do the 4(i)???

 

[areeba240]

how to do (iii)

 

[Thought blocker]

View attachment 61598
how to do (iii)

Here you go.

 

[areeba240]

Here you go.

Here you go.

thanks alot

 

[krishnapatelzz]

https://www.xtremepapers.com/community/threads/maths-statistics-and-pure-maths-videos.46182/

 

[krishnapatelzz]

https://www.xtremepapers.com/community/threads/how-tough-are-the-chapters-of-statistics-1.46186/

 

[krishnapatelzz]

https://www.xtremepapers.com/community/threads/suggestion-for-9709-mechanics-p4-5.46187/
mechanics !!!!!!!!!!!!!

 

[i_try9621]

Need help with Q19

 

[DragonCub]

View attachment 61648 Need help with Q19

 

[FrostBite92]

Can anyone help with this? Can't wrap my head around this problem.

A staff car park at a school has 13 parking spaces in a row. There are 9 cars to be parked.
i. How many Different arrangements are there for parking the 9 cars and leaving 4 empty spaces?
ii.How many different arrangements are there if the 4 empty spaces are next to each other?
iii. if the parking is random, find the probability that there will not be 4 empty spaces next to each other.

Any help would be appreciated.

 

[i_try9621]

View attachment 61651
View attachment 61652

View attachment 61653

In i, Why do we need two points that are on both planes? Also couldn't it be that the planes were skewed?