- Messages
- 20
- Reaction score
- 2
- Points
- 3
i want 2012 november plshttp://papers.xtremepapers.com/CIE/Cambridge International A and AS Level/Mathematics (9709)/9709_s12_qp_31.pdf
someone please help me with no 8
thanks in advance
We are currently struggling to cover the operational costs of Xtremepapers, as a result we might have to shut this website down. Please donate if we have helped you and help make a difference in other students' lives!
Click here to Donate Now (View Announcement)
i want 2012 november plshttp://papers.xtremepapers.com/CIE/Cambridge International A and AS Level/Mathematics (9709)/9709_s12_qp_31.pdf
someone please help me with no 8
thanks in advance
can u pls give me 2012 nov cie as and alevel maths p1 p4 s1 s2 plsyou're welcome
Herecan u pls give me 2012 nov cie as and alevel maths p1 p4 s1 s2 pls
assalamualaikum! i have a question, it's a very old one (june 89), please help me!
The equation y= ax^2 - 2bx + c , where a,b and c are constants, with a>0.
a) Find, in terms of a,b and c the coordinates of the turning point on the curve.
b) Given that the turning point of the curve lies on the line y=x, find an expression for c in terms of a and b. Show that, in this case, whatever the value of b, c> -(1/4a).
it's the "show that" that i'm getting difficulty
jazakallah!
jazakallahu khairan!!!!Walaikum AsSalam Warahmatullahi Wabarakatohu
a) dy/dx = 2ax - 2b
2ax - 2b = 0
ax - b = 0
x = b/a
Put this in eq and get the y-coordinate:
y = a(b/a)^2 - 2b(b/a) + c
y = b^2/a - 2b^2/a + c
y = -b^2/a + c
b) Vertex lies on the line y = x, so we can form an equation using the coordinates from (a)
b/a = -b^2/a + c
c = (b^2 + b)/a
Now you have to treat this equation as an entirely different function. The methods available are completing square or finding discriminant.
Completing square:
c = 1/a (b^2 + b + (1/2)^2 - (1/2)^2)
c = [1/a (b + 1/2)^2] - 1/4a
The y-coordinate of the vertex is 1/4a. We know that the graph is a U-shaped parabola so the range is c >= -1/4a.
Using discriminant:
b^2 + b - ac = 0
D = 1 - 4(1)(-ac)
For real roots, D>=0
1 + 4ac >=0
c >= -1/4a
My answer includes the the equality symbol but i am sure you made a typo there.
IS THIS IN AS? OR IS IT A2assalamualaikum! i have a question, it's a very old one (june 89), please help me!
The equation y= ax^2 - 2bx + c , where a,b and c are constants, with a>0.
a) Find, in terms of a,b and c the coordinates of the turning point on the curve.
b) Given that the turning point of the curve lies on the line y=x, find an expression for c in terms of a and b. Show that, in this case, whatever the value of b, c> -(1/4a).
it's the "show that" that i'm getting difficulty
jazakallah!
it is maths A-levelIS THIS IN AS? OR IS IT A2
I need help with Q 10(v) 9709_w06_qp_1
Wa iyyakum!!jazakallahu khairan!!!!
but could u please elaborate a little more...? i didnt really understand how u did the discriminant part.
thanks a lot!Ok, you have to get that A keeps moving up for a certain length of time even after B hits the ground owing to it's velocity at the time B comes to rest (Newton's first law). This is when the string gets slack. A then drops down from the max. height for the same length of time until the string gets taught again and stops it's movement.
So, first you have to find the velocity with which B hit the ground. Use the eq. v= u +at, initial velocity of B was 0 as it was at rest, the acceleration is the same as you calculated in part i and the time is 1.6 sec. The velocity of A is the same as B at the instant B hit the ground.
Now, you have to calculate the time it takes for A to come to rest. Use the same eq as before but take acceleration as -9.8 because gravity is the only force acting on it now. Final velocity is 0 and initial is the one you calculated. Then double this time to find your answer.
Hope this Helps!
http://papers.xtremepapers.com/CIE/Cambridge International A and AS Level/Mathematics (9709)/9709_w08_qp_1.pdf
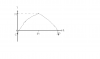
Please help me with no. 5 (iii).. I do not understand trigonometric graphs completely!
An explanation will be appreciated.
Thanks
Mechanicsss


q10 b ii) P33 S12
It's only one mark, but I just can't work it outHelp anybody?
10
(b) (i) On a sketch of an Argand diagram, shade the region whose points represent complex
numbers satisfying the inequalities |z − 2 + 2i| ≤ 2,
arg z ≤ −1/4π and Re z ≥1, where Re z denotes the real part of z.
(ii) Calculate the greatest possible value of Re z for points lying in the shaded region
I can draw the argand diagram easily but how are we supposed to work out the last part?

For almost 10 years, the site XtremePapers has been trying very hard to serve its users.
However, we are now struggling to cover its operational costs due to unforeseen circumstances. If we helped you in any way, kindly contribute and be the part of this effort. No act of kindness, no matter how small, is ever wasted.
Click here to Donate Now