- Messages
- 9
- Reaction score
- 1
- Points
- 3
We are currently struggling to cover the operational costs of Xtremepapers, as a result we might have to shut this website down. Please donate if we have helped you and help make a difference in other students' lives!
Click here to Donate Now (View Announcement)
I think you've confused some numbering.....PURE MATHS 1 FEB/MARCH ANSWERS (MINE)
1) P=6
2) f(x)=2/9x^3-x^2+2
3)118 cm^2
4)dy/dx=-2(2x-1)^-2 +2
d^2y/dx^2=8(2x-1)^-3
5) x=8 in minimum and x=1 is the maximum
6) a) q= 2 or -11/3 b) 130.7
7) b) p=8 and n=42
8) 54.8 and 125.3 b)sq root 3 +tan (2x)
9) a) k=2 b) f inverse is 2- square root (x-3) and domain is f(x) >4 c) gf(x) range is < or equal to 1/3 (I confused the most here tho)
10) 117pi/4 b) (0,78/11)
11)a)8 square root 2, b) T(4,8)
Please share your answers if you solved,
4) I wrote an 8 instead of 0 by mistake so I took the wrong values, yes your answer is correct.I think you've confused some numbering.....
I've got some different answers.
For 4 ii) x= 0 max and x=1 min
One of the angles in 7 a) is 54.7° not 54.8° according to me...a little difference though but the answer mark will be lost
For 7 b) my a is same but the value of b is -4
For 8 iv) my range is 0<gf(x)<1
And plz tell me the ans of the very last part i.e. 10 iii) mine is (4.5, 7.5)
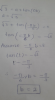 If you actually try -4 you'll get y=0, but I think you took 2/3pi which is wrong because t (in my pic) value has to be on the negative side so you should take -pie/3 (you're not shifting it towards left or right ), maybe they'll accept -4 but its not in the domain range they've given us.
If you actually try -4 you'll get y=0, but I think you took 2/3pi which is wrong because t (in my pic) value has to be on the negative side so you should take -pie/3 (you're not shifting it towards left or right ), maybe they'll accept -4 but its not in the domain range they've given us. Oh yes I've looked into it your value for b is correct4) I wrote an 8 instead of 0 by mistake so I took the wrong values, yes your answer is correct.
7) I think I got it 54.8 roundings it to 3sf but I really don't remember tbh,
and I got b=2 View attachment 64678 If you actually try -4 you won't get y=0, I think you took 2/3pi which is wrong because your x value has to be on the negative side so you should take -pie/3 (you're not shifting it towards left or right )
8) for iv I confused somewhere I understood the range of f(x) has to be greater than 1 but I can't figure how to progress so please help
and 10)iii yes its (9/2, 15/2) sorry I messed up the order
Yes, that's the thing, I think your answer is correct tho,Oh yes I've looked into it your value for b is correct
For 8 iv) first see the domain of the inner function f(x) which is x<2 and then its range for this domain which is f(x)>3
Now the range of f will be domain of g and according to this domain the range of g(x) will be the range of gf(x). From here the answer 0<gfx<1 comes
But I was a bit confused as what to use as the domain of f(x). x<2 or x<1
Alright thanks!!Yes, that's the thing, I think your answer is correct tho,
I'll ask my mom's friend to help out so we could be sure, and I'll inform you
You can find them at gceguide.comanyone who can give me recent topical past papers for p1 and s1
Heey I still haven't got a reply from my moms' friend but I was thinking that I think the question is pretty simple like they said domain has to be greater than 1, therefore it can't be 0, and actually we were confused with what domain to take but since they said x>1 and we know f(x) range will be the domain of g(x) so if we place f(x)>1 you won't get a value for it except when f(x)>3, therefore, its obvious to say that x can only be 3 and up to infinity, and if you try values greater than 3 you'll find it tends towards 0, so I think its right to say 0<gf(x)<1,You can find them at gceguide.com
It's less than 1 not greater...if we use x<1 then the range would be 0<gfx<2/3Heey I still haven't got a reply from my moms' friend but I was thinking that I think the question is pretty simple like they said domain has to be greater than 1, therefore it can't be 0, and actually we were confused with what domain to take but since they said x>1 and we know f(x) range will be the domain of g(x) so if we place f(x)>1 you won't get a value for it except when f(x)>3, therefore, its obvious to say that x can only be 3 and up to infinity, and if you try values greater than 3 you'll find it tends towards 0, so I think its right to say 0<gf(x)≤1,
I gave it a thought its actually a reciprocal standard formuala (a/x-h)+k , so if we take 2/x-1 then this means the asymptotes are x=1 and y=0, and for x>1 the range has to be what I mentioned before,
does it make sense?
No we have to use the domain of f(x)
Yo they're saying the domain of g(x) has to be x>1, f(x) range is the domain of g(x), in other words, f(x)>1No we have to use the domain of f(x)
Here I've got the answersYo they're saying the domain of g(x) has to be x>1, f(x) range is the domain of g(x), in other words, f(x)>1
f(x) can't be >1 but can be greater than 3 based on completed sq.form, therefore the range of gf(x) is 0<gf(x)<1
why are they taking the domain of gf(x) to be lesser than 1?Here I've got the answers
For almost 10 years, the site XtremePapers has been trying very hard to serve its users.
However, we are now struggling to cover its operational costs due to unforeseen circumstances. If we helped you in any way, kindly contribute and be the part of this effort. No act of kindness, no matter how small, is ever wasted.
Click here to Donate Now