Physics: Post your doubts here!

[Xylferion]

MJ 11 P11 Question number 27. It's quite difficult I think so anybody wanna try?

It's a tricky question in that you need to understand how orders behave around the central maxima.

A diffraction grating with 500 lines per mm, with light of wavelength 600 nm passing through it.

For diffraction gratings, there are two formulas you always need to known. The first being: d sin θ = n λ.
d being the width of each slit.
theta being the angle between the central maxima and each order.
n being the number of orders.
and λ being the wavelength.

The other one deals with finding out the spacing of each slit, from the number of lines there are.
If there are 100 lines, the spacing for 1 slit will be 1/100.

This gives us the formula, d = 1/N, with N being the number of lines.

With all the info they have already given us, we can first find out how many orders there are, in order to determine the number of images produced.

They tell us, 500 lines " per " mm. The number of lines present will be per metre, so we convert the mm to metres. This gives us 500 / 1*10^-3 m
Which is 500,000 lines.

Plug this into d = 1/N to find the split spacing, which is 1/500,000 = 2 x 10^-6 m

Now before we go any further, we need to know, that the image produced at Y, is along the central maxima. Which follows an order of n = 0.
They mention that X and Z are "parallel" to the grating, this means that the angle at which they are being viewed at is 90 degrees.

That gives us,
2 x 10^-6 ( Sin 90 ) = n ( 600 x10^-9)
Since Sin 90 = 1,
n = 2 x 10^-6 / 600 x 10^-9
n = 3.333

3.333 might seem like an odd number to work with, but it is completely fine, since 3.33 still belongs to the 3rd order.
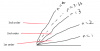
Hence we consider there to be 3 orders from the central maxima.

Now as for the number of images produced, there are 3 orders, on BOTH sides of the central maxima. That is 3 orders below n = 0, and 3 orders above n = 0.

That is a total of 7 images produced as he moves his head from X to Z, since the central maxima AKA "Y", is where he would also see an image.
They did mention " different " angles, along X to Z, that is how you are able to know that his head need not be at X and Z only.

With 7 images being produced, your only answer will be D.

Hope that helped

 

[Physux]

http://maxpapers.com/wp-content/uploads/2012/11/9702_s13_qp_22.pdf
Need help with question 2b. While taking acceleration why will we not add the weight and the resultant to calculate the total force and then calculate acceleration. The graph gives resultant only which means that the weight which is balanced needs to be considered as well. So why don't we add both the forces and then take acceleration? Help asap please.

 

[The Sarcastic Retard]

http://maxpapers.com/wp-content/uploads/2012/11/9702_s13_qp_22.pdf
Need help with question 2b. While taking acceleration why will we not add the weight and the resultant to calculate the total force and then calculate acceleration. The graph gives resultant only which means that the weight which is balanced needs to be considered as well. So why don't we add both the forces and then take acceleration? Help asap please.

http://physics-ref.blogspot.in/2014/06/9702-june-2013-paper-22-worked.html

 

[Xylferion]

http://maxpapers.com/wp-content/uploads/2012/11/9702_s13_qp_22.pdf
Need help with question 2b. While taking acceleration why will we not add the weight and the resultant to calculate the total force and then calculate acceleration. The graph gives resultant only which means that the weight which is balanced needs to be considered as well. So why don't we add both the forces and then take acceleration? Help asap please.

The resultant force is what its name indicates. It is the result of a difference between two forces and the directions in which they act. It can be a little confusing since they did not shed light on how they got the resultant force but the fact of the matter is, adding a resultant force to one of the forces that "probably" defined it, would serve no purpose as there would be no reason to consider it a resultant force then.

Try to think of the resultant force as a product of two forces, one of which might have included the weight. They did not indicate to you at all that you're required to consider the weight, so don't bother much with it. Simply use what's given to you.

Hope all of that made sense

 

[Physux]

The resultant force is what its name indicates. It is the result of a difference between two forces and the directions in which they act. It can be a little confusing since they did not shed light on how they got the resultant force but the fact of the matter is, adding a resultant force to one of the forces that "probably" defined it, would serve no purpose as there would be no reason to consider it a resultant force then.

Try to think of the resultant force as a product of two forces, one of which might have included the weight. They did not indicate to you at all that you're required to consider the weight, so don't bother much with it. Simply use what's given to you.

Hope all of that made sense

I get that but see resultant is something you would only get once the weight is balanced. Like that's the extra force which is making it accelerate. This is confusing. :/

 

[Physux]

SOMEONE !!!

Is the answer for the projectile one B? And the density question D?

 

[Physux]

How to calculate the angles?

 

[RahatMT]

can someone solve the first question of variant 2/o/n/ 2014 paper 2 and show me your working

 

[Xylferion]

I get that but see resultant is something you would only get once the weight is balanced. Like that's the extra force which is making it accelerate. This is confusing. :/

No. It's not necessary for the weight to be balanced. The object could be on a slope for all you know with a force acting on or at it.

If the weight is balanced, the body is in equilibrium, the resultant force would be zero, but that is clearly not the case here.

Balanced forces do not give a resultant force O.O, 5 to the right balanced by 5 to the left, does NOT give a resultant force in any direction.

They did not say that the weight is responsible for the acceleration; and it would be wrong to assume so as we also need to take into consideration the platform, a slope or not.

Hope that made sense

 

[Physux]

View attachment 51963

Is that A?

 

[Physux]

No. It's not necessary for the weight to be balanced. The object could be on a slope for all you know with a force acting on or at it.

If the weight is balanced, the body is in equilibrium, the resultant force would be zero, but that is clearly not the case here.

Balanced forces do not give a resultant force O.O, 5 to the right balanced by 5 to the left, does NOT give a resultant force in any direction.

They did not say that the weight is responsible for the acceleration; and it would be wrong to assume so as we also need to take into consideration the platform, a slope or not.

Hope that made sense

I meant that the weight is balanced by some force first and only then the resultant force produces acceleration. I think you misinterpreted or maybe I didn't convey it properly.

 

[Physux]

can someone solve the first question of variant 2/o/n/ 2014 paper 2 and show me your working

Are you sure about this? Post the paper link please?

 

[fantastic girl]

Assalamu Alaikum.
http://studyguide.pk/Past Papers/CIE/International A And AS Level/9702 - Physics/9702_w10_qp_41.pdf
Can someone tell me how do we get a +240 for P to Q in Q2 b(iii) :/
i dont get it....see the final volume is less then initial so how can work done be positive ? :S

 

[fantastic girl]

check at
http://physics-ref.blogspot.com/2014/09/9702-november-2010-paper-41-42-worked.html

its shown as
Work done = pΔV = (4.0x105)(6x10-4) = 240J
how can it be 6x10^-4 ?
shudnt it be 2x10^-4 - 8x10^-4 = -6x10^-4
its final - initial ryt?

 

[amal sharkawi]

Can anyone answer this plz
Why the answer is D ????

 

[fantastic girl]

we take the magnitude
and then we understand it by either work done BY gas or work done ON gas

i dont get it bro
why cnt i use the formula ? :/
isnt the work being done ON the system here ?

 

[Xylferion]

How to calculate the angles?

The question is about diffraction gratings, so you need to recall the formula d sin theta = n lambda

d is the slit separation, which in this question it is 2.00 micrometers giving 2.00 x 10^-6 meters.
sin theta is what you're going to use to find the angle between the orders.
n = order number
lambda = wavelength

To find the angle separation, you subtract the angle for the first order from the angle of the second order.

For the first angle,
2.00 x 10^-6 ( Sin theta ) = 1 ( 600 x 10^-9)
Sin theta = 0.3
Theta = Sin^-1 ( 0.3)
Theta = 17.45

For the second angle,
2.00 x 19^-6 ( Sin theta ) = 2 ( 600 x 10^-9)
Sin theta = 0.6
theta = Sin^-1 ( 0.6)
theta = 36.86

Angle separation = 36.86 - 17.45 = 19.4(1)

 

[fantastic girl]

we are using the formula, but are only taking the magnitude

owkay.
can u tell me why we arent taking the sign into consideration?

 

[Xylferion]

can someone solve the first question of variant 2/o/n/ 2014 paper 2 and show me your working

Not sure if you want the whole of question 1, but I'll just explain the first part for now.

For part (a),

The Young's modulus of a metal wire is 1.8 × 10^11 Pa
Young's modulus is defined as stress/ strain.
You're given the strain which is 8.2 × 10^–4.

They want the stress, in giga pascals. giga being 10^9

First find the stress, this will be 1.8 x 10^11 * 8.2 x 10^-4.
Which is 147,600,000 Pascals.

1 pascal = 10^-9 giga pascals since it's / 10^-9.
147,600,00 pascals = 0.1476 GPa.
You can round this up if you want, to either 0.15 or 0.148. I'd recommend 3 significant figures.

Hope that helped

 

[fantastic girl]

it's already said work done ON gas.
+work done is energy and is a scalar.
a negative energy has on meaning

oh
thanks alot