Physics: Post your doubts here!

[Instigator]

12.
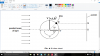
Take moments about the bottom left corner
clockwise = aniti-clockwise moments
Fh + Wa = 2Wa

21.
Strain energy is the area under the graph
Break the graph into sections and calculate the area
Remember to multiply the length by 10^-2 since it's in cm

thanks a bunch 21 had me confused

 

[Maayee]

http://papers.xtremepapers.com/CIE/...and AS Level/Physics (9702)/9702_s08_qp_4.pdf
can someone help me with Q5 b

 

[ashcull14]

 

[ashcull14]

see solution 26 at
http://physics-ref.blogspot.com/2014/10/physics-9702-doubts-help-page-4.html

thanks ..im having a problem in finding the area under the line of the graph ..how did u count the boxes?

 

[Zoha Ali]

http://papers.xtremepapers.com/CIE/...nd AS Level/Physics (9702)/9702_w12_qp_11.pdf

Can anyone please explain question 11?

 

[The Sarcastic Retard]

http://papers.xtremepapers.com/CIE/Cambridge International A and AS Level/Physics (9702)/9702_w12_qp_11.pdf

Can anyone please explain question 11?

Momentum of whole system is conserved not of an object.

 

[nehaoscar]

9093 English AS
Any tips on how to write a commentary?
Like the layout and perhaps a list of features and effects to look for?
Can anyone provide me with sample commentaries if you have done in school? (preferably with marks)

Also tips on paper 1 and paper 2 as well to get an A
Thanks in advance

 

[***amd***]

http://papers.xtremepapers.com/CIE/...nd AS Level/Physics (9702)/9702_s15_qp_11.pdf
Q19 and Q38 ?

 

[Akshajistari]

These ones please?!!

 

[Rizwan Javed]

View attachment 57751 View attachment 57752 View attachment 57753 View attachment 57754 These ones please?!!

13. Taking moments about the pivot, and apply the principle of moments:

(taking all moments in anti-clockwise direction to be positive)

sum of all anti-clockwise moments - sum of all clockwise moments = 0

* 'd' is the distance from the pivot where the 50g weight should be hung.
* Also I have converted all the lengths to meters (m)

(20/1000 * 9.81 * 60/100) - (10/100 * 100/1000 * 9.81) + (50/1000 * 9.81 * d) = 0
by solving it we get:

d = - 0.04m = -4 cm

the minus sign here indicates that the moment produced by the 50g weight is in the clockwise direction. Hence the 50g weight should be placed 4cm on the right side of the pivot in order to achieve this. So the mark at which it'll be placed is 44cm (40+4)

The Answer is therefore C.

 

[Rizwan Javed]

14. Let the height from which it is dropped be 'h'.

First, consider the sphere before colliding:
it hits the metal plate with speed 'u' that means that it's final speed after release from rest is 'u'.

so find 'h' in terms of 'u' by applying equation of motion:

vf^2 = vi^2 + 2as (vf = final velocity; vi = initial velocity)
putting the values:
(u)^2 = (o)^2 + 2gh
u^2 / 2g = h ---- (1)

Now consider the sphere after it has jumped off after colliding with the metal plate.
It leaves the surface with speed 'v' that means it leaves the metal plate with K.E = 1/2 mv^2
apply the law of conservation of energy:
K.E lost = Gain in P.E
(i'm letting the mass of sphere to be 'm')
1/2 mv^2 = m g (h/2)
h = v^2 /g --- (2)

put these two equations equal and solve to get v/u.
u^2 / 2g = v^2 / g
v^2 / u^2 = 1/2
v/u = 1/√2

So the answer is C.

 

[Akshajistari]

14. Let the height from which it is dropped be 'h'.

First, consider the sphere before colliding:
it hits the metal plate with speed 'u' that means that it's final speed after release from rest is 'u'.

so find 'h' in terms of 'u' by applying equation of motion:

vf^2 = vi^2 + 2as (vf = final velocity; vi = initial velocity)
putting the values:
(u)^2 = (o)^2 + 2gh
u^2 / 2g = h ---- (1)

Now consider the sphere after it has jumped off after colliding with the metal plate.
It leaves the surface with speed 'v' that means it leaves the metal plate with K.E = 1/2 mv^2
apply the law of conservation of energy:
K.E lost = Gain in P.E
(i'm letting the mass of sphere to be 'm')
1/2 mv^2 = m g (h/2)
h = v^2 /g --- (2)

put these two equations equal and solve to get v/u.
u^2 / 2g = v^2 / g
v^2 / u^2 = 1/2
v/u = 1/√2

So the answer is C.

Thank You so much for those two!



Any takers for the others?

 

[Rizwan Javed]

Thank You so much for those two!



Any takers for the others?

I might have solved the other two as well but i haven't studied those topics yet so maybe anyone else can help Dark Destination @F.M.Z. 7 cool Hassan

 

[The Sarcastic Retard]

Q1, Q2a, Q4

 

[The Sarcastic Retard]

Q1, 2a, Q4

 

[Sarosh Jameel]

Plz help me with part (II) .. its june 09

 

[The Sarcastic Retard]

Plz help me with part (II) .. its june 09

Let theta = @
Let omega = w

SQ is clearly r(sin@)
So SQ = rsin(@/t)*t Where @/t = w

 

[Sarosh Jameel]

t

Let theta = @
Let omega = w
View attachment 57781
SQ is clearly r(sin@)
So SQ = rsin(@/t)*t Where @/t = w

thanks

 

[Sarosh Jameel]

PLZ help with this !

 

[The Sarcastic Retard]

PLZ help with this !View attachment 57783

a)
When the stone will be at bottom most point, the Weight will act vertically downwards and reaction force (force excerted by glue on rod) towards the center of circle which is vertically upwards.
b)
T - W = mrw^2
15 = 0.3(0.85)w^2
w = 7.6rad/s