- Messages
- 51
- Reaction score
- 3
- Points
- 8
We are currently struggling to cover the operational costs of Xtremepapers, as a result we might have to shut this website down. Please donate if we have helped you and help make a difference in other students' lives!
Click here to Donate Now (View Announcement)
w to w^2 is the diameterhow did you find the radius?
Q8(i) I have done this before in this thread, searchThank you So Much!!!!!!!!!!!!!!
If possible can u help me with my May june 2008 doubts..on Argand diagrams
http://www.xtremepapers.com/CIE/International A And AS Level/9709 - Mathematics/9709_s08_qp_3.pdfPlease help me with the 8th one..part 1 and the 5th one part 2!!!!!
Please!!
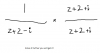
Post the links!in ON11 P31, Q1, we found x using 'ln'. What i want to know is if there is a constant on one side do i take it as ln3 or lne^3, In this question they took ln3 while in other questions for eg, differential equations we take it as lne^3. Please Help.
This question, how can i solve it here means writing the powers down and all not too much symbols or space!
This question, how can i solve it here means writing the powers down and all not too much symbols or space!
Wait i'll tell you in afternoon after i solve this paper myself!is it possible for you to post the solution? or if you could tell the steps?
e^x = 1 + 6e^−x
Wait i'll tell you in afternoon after i solve this paper myself!
hey could you please help me with my doubt.I've posted it twice here but haven't gotten any reply.Since you've been helping a lot of people,I am asking you.e^x = 1 + 6e^−x
Using u = e^x
The equation becomes
u = 1 + 6/u
u^2 = u + 6
u^2 - u - 6 = 0
(u+2)(u-3) = 0
u = -2 or u = 3
e^x = -2
This cannot be solved as ln or lg of a negative number is undefined!
The only solution is
e^x = 3
x = ln3 / lne
x = ln3
x = 1.10
We take lne^x
but according to power rule, the power becomes coefficient
so lne^x = xlne
and lne = 1
so
xlne = x
See the notes i posted on logs above !
For almost 10 years, the site XtremePapers has been trying very hard to serve its users.
However, we are now struggling to cover its operational costs due to unforeseen circumstances. If we helped you in any way, kindly contribute and be the part of this effort. No act of kindness, no matter how small, is ever wasted.
Click here to Donate Now