Physics: Post your doubts here!

[redox233]

CAN ANYONE HELP ME SOLVE THIS QUESTION?

 

[PlanetMaster]

View attachment 70291

CAN ANYONE HELP ME SOLVE THIS QUESTION?

Since this is an MCQ, lets first do a quick sanity check. The cube is floating in equilibrium in mercury so the density of mercury should be heaver than the cube itself i.e the answer is either C or D.
Now, the cube floats 42% above the surface and therefore the immersed part i.e. the part that displaces mercury is 100-42% = 58% or 0.58.

Now we can have p1/v1 = p2/v2 where
p1 is the density of mercury,
p2 is the density of iron,
and therefore p1 = p2(v1/v2)
This gives us p1 = 7900/0.58 = 13,621 kg/m^3

 

[redox233]

Since this is an MCQ, lets first do a quick sanity check. The cube is floating in equilibrium in mercury so the density of mercury should be heaver than the cube itself i.e the answer is either C or D.
Now, the cube floats 42% above the surface and therefore the immersed part i.e. the part that displaces mercury is 100-42% = 58% or 0.58.

Now we can have p1/v1 = p2/v2 where
p1 is the density of mercury,
p2 is the density of iron,
and therefore p1 = p2(v1/v2)
This gives us p1 = 7900/0.58 = 13,621 kg/m^3

thankyou but can you please tell which formula is p1v1=p2v2 and what do v1 and v2 signify here?

 

[PlanetMaster]

thankyou but can you please tell which formula is p1v1=p2v2 and what do v1 and v2 signify here?

Since this is a MCQ question, I skipped a lot of steps and used just ratios and the ratio based on simply density=mass/vol. This is where p1v1=p2v2 comes from.

To elaborate the steps, lets first consider the immersed part where weight of solid equals the weight of liquid displaced.
[imath]W_{s}=W_{ld}[/imath]
And since w=mg, we get
[imath]m_{s}g=m_{ld}*g[/imath]\
And since density=mass/vol, we get
[imath]P_{s}V_{s}=P_{ld}V_{ld}[/imath] as g cancels out
Now volume of liquid displaced is equal to the volume of the immersed portion, so lets write it as
[imath]P_{s}V_{s}=P_{ld}V_{imm}[/imath]
Now we rearrange as
[imath]\frac{P_{s}}{P_{l}}=\frac{V_{imm}}{V_{s}}=0.58[/imath]
And so
[imath]P_{l}=\frac{P_{s}}{0.58}=\frac{7900}{0.58}=13621\:kg\:m\:^{-3}[/imath]

Hope that helps!

 

[redox233]

Since this is a MCQ question, I skipped a lot of steps and used just ratios and the ratio based on simply density=mass/vol. This is where p1v1=p2v2 comes from.

To elaborate the steps, lets first consider the immersed part where weight of solid equals the weight of liquid displaced.
[imath]W_{s}=W_{ld}[/imath]
And since w=mg, we get
[imath]m_{s}g=m_{ld}*g[/imath]\
And since density=mass/vol, we get
[imath]P_{s}V_{s}=P_{ld}V_{ld}[/imath] as g cancels out
Now volume of liquid displaced is equal to the volume of the immersed portion, so lets write it as
[imath]P_{s}V_{s}=P_{ld}V_{imm}[/imath]
Now we rearrange as
[imath]\frac{P_{s}}{P_{l}}=\frac{V_{imm}}{V_{s}}=0.58[/imath]
And so
[imath]P_{l}=\frac{P_{s}}{0.58}=\frac{7900}{0.58}=13621\:kg\:m\:^{-3}[/imath]

Hope that helps!

thankyou so much

 

[redox233]

CAN SOMEONE PLEASE EXPLAIN THIS QUESTION. THE ANSWER IS D

 

[PlanetMaster]

View attachment 70388

CAN SOMEONE PLEASE EXPLAIN THIS QUESTION. THE ANSWER IS D

Resistance of wire [imath]R = \frac{ρL}{A}[/imath]
And cross sectional area [imath]A = \frac{πd^{2}}{4}[/imath]

When the wire is stretched, the diameter is reduced to 94%.
This causes the area of stretched wire to be
[imath]A_{s} = \frac{π(0.94d)^{2}}{4} = 0.8836(\frac{πd^{2}}{4})[/imath] = 0.8836A

Volume of the wire is cross-sectional area x length,
[imath]V = AL[/imath]
And since the volume of the wire is unchanged,
[imath]AL=A_{s}L_{s}[/imath]
and the diameter is reduced to 94%, the length has to be
[imath](\frac{πd^{2}}{4})L=(\frac{π(0.94d^{2})}{4})(L_{s})[/imath]
[imath]L_{s}=\frac{L}{0.94^{2}}[/imath]

So, the length of the stretched wire is [imath]\frac{L}{0.94^{2}}[/imath] or [imath]1.132L[/imath]

Resistance of wire [imath]R = \frac{ρL}{A}[/imath]

So Resistance of stretched wire
[imath]R_{s} = \frac{ρL_{s}}{A_{s}} = \frac{ρ(1.132L)}{0.8836A} = 1.28\frac{ρL}{A} = 1.28R[/imath]

Hope this explains!

 

[redox233]

tysm

 

[redox233]

Resistance of wire [imath]R = \frac{ρL}{A}[/imath]
And cross sectional area [imath]A = \frac{πd^{2}}{4}[/imath]

When the wire is stretched, the diameter is reduced to 94%.
This causes the area of stretched wire to be
[imath]A_{s} = \frac{π(0.94d)^{2}}{4} = 0.8836(\frac{πd^{2}}{4})[/imath] = 0.8836A

Volume of the wire is cross-sectional area x length,
[imath]V = AL[/imath]
And since the volume of the wire is unchanged,
[imath]AL=A_{s}L_{s}[/imath]
and the diameter is reduced to 94%, the length has to be
[imath](\frac{πd^{2}}{4})L=(\frac{π(0.94d^{2})}{4})(L_{s})[/imath]
[imath]L_{s}=\frac{L}{0.94^{2}}[/imath]

So, the length of the stretched wire is [imath]\frac{L}{0.94^{2}}[/imath] or [imath]1.132L[/imath]

Resistance of wire [imath]R = \frac{ρL}{A}[/imath]

So Resistance of stretched wire
[imath]R_{s} = \frac{ρL_{s}}{A_{s}} = \frac{ρ(1.132L)}{0.8836A} = 1.28\frac{ρL}{A} = 1.28R[/imath]

Hope this explains!

wont we subtract 0.94 from 1D as it says DECREASED BY 94%

 

[PlanetMaster]

wont


wont we subtract 0.94 from 1D as it says DECREASED BY 94%

It says decreases to 94%!
If it were decreases by 94%, then we would subtract 0.94 from 1.

 

[redox233]

CAN SOMEONE EXPLAIN THIS QUESTION?

 

[redox233]

AND THIS QUESTION AS WELL THANKYOU. HOW WILL WE FIND THE AMPLITUDE IN THIS QUESTION?

 

[PlanetMaster]

View attachment 70518
CAN SOMEONE EXPLAIN THIS QUESTION?

You need to take intersections of both waves where they are going in the same direction.
From the graph, these can be either 0 to 0.5 or it can be 0.5 to 0.8.

We can then use ratios to calculate the phase difference
[imath]\frac{\Delta t}{T} = \frac{\Delta \phi}{360^{\circ}}[/imath]

Since T is 0.8 i.e one full cycle, using [imath]\Delta t = 0.3[/imath] gives us [imath]\Delta \phi = 135^{\circ}[/imath] and using [imath]\Delta t = 0.5[/imath] gives us [imath]\Delta \phi = 225^{\circ}[/imath]

So the answer is C i.e 135 which is also 360-225 i.e. 135

 

[PlanetMaster]

View attachment 70537

AND THIS QUESTION AS WELL THANKYOU. HOW WILL WE FIND THE AMPLITUDE IN THIS QUESTION?

During one oscillation, point A moves a distance of 80mm as given in the question.
And during this one oscillation, point A would return to its original position. Any point would move a distance = 4 time the amplitude during one oscillation, .

Remember, as defined above, the displacement is up and down while the wave travels to the right (in this case). Amplitude is the maximum displacement form the equilibrium position.

Consider the point A for example, which is originally on the minima. During one oscillation, it will move
1. a distance a from its current position at A to the equilibrium position,
2. another distance a from the equilibrium position to reach the maxima (crest),
3. another distance a to move from the maxima (crest) back to reach the equilibrium position again,
4. and finally, another distance a from the equilibrium position to return to its original position at A.

Thus, amplitude = 80/4 = 20mm

 

[redox233]

Sorry to disturb you again and again but can you pls tell that how the graphs are intersecting at 0.5 and 0.8

You need to take intersections of both waves where they are going in the same direction.
From the graph, these can be either 0 to 0.5 or it can be 0.5 to 0.8.

We can then use ratios to calculate the phase difference
[imath]\frac{\Delta t}{T} = \frac{\Delta \phi}{360^{\circ}}[/imath]

Since T is 0.8 i.e one full cycle, using [imath]\Delta t = 0.3[/imath] gives us [imath]\Delta \phi = 135^{\circ}[/imath] and using [imath]\Delta t = 0.5[/imath] gives us [imath]\Delta \phi = 225^{\circ}[/imath]

So the answer is C i.e 135 which is also 360-225 i.e. 13

 

[PlanetMaster]

Sorry to disturb you again and again but can you pls tell that how the graphs are intersecting at 0.5 and 0.8

No worries pal. For both waves, we can take the points they intersect 0 displacement in the same direction.

Like this:

 

[redox233]

PlanetMaster can u pls explain this uestion? thankyou!

 

[Lemniscates]

PlanetMaster can u pls explain this uestion? thankyou!

Hey redox233, I am not sure if you still need help on this, but let me explain this to you:-

[imath]\rightarrow[/imath] This is a classic problem of standing waves.
[imath]\rightarrow[/imath] Given that the lowest sound produced is [imath]92[/imath] Hz, such as a node at the mouthpiece and an antinode at the other open end.

Hence, this is what we have,



We know that distance between a node and an antinode is [imath]\lambda/4[/imath].

[imath]\therefore \lambda/4[/imath] corresponds to [imath]92 \space \mathrm{Hz}[/imath].

The next arrangement of the standing wave to produce another frequency is as follows,



Here, we have [imath]\lambda/2 + \lambda/4 = 3\lambda/4[/imath]

[imath]\therefore 3\lambda/4[/imath] corresponds to [imath]3 \times \lambda/4 = 3 \times 92 =[/imath] [imath]276 \space \mathrm{Hz}[/imath]

Here is the next arrangement and you can follow this idea to get the overall correct answer to this question,



Here, we have [imath]\lambda + \lambda/4 = 5\lambda/4[/imath]

[imath]\therefore 5\lambda/4[/imath] corresponds to [imath]5 \times \lambda/4 = 5 \times 92 =[/imath] [imath]460 \space \mathrm{Hz}[/imath]

[imath]\rarr[/imath] If you simply look at all the options now in the question, we can clearly see that C seems to be the correct option.
[imath]\rarr[/imath] However, feel free to visualize the next arrangement and its corresponding frequency and match it with option C.

I hope this helps.
Good luck!

 

[Lemniscates]

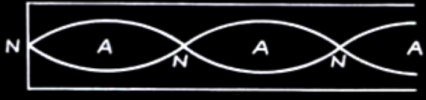
No worries pal. For both waves, we can take the points they intersect 0 displacement in the same direction.

Like this:View attachment 70545

Hey PlanetMaster,

What software do you use to create such graphs that go with the background of the XPC theme? 🤔
Also, it would be great if you could provide a Math editor in the options so that we don't have to externally use LaTeX to write Mathematical equations here.

Thank you! ^_^

 

[unknownerror21]

a child of mass 35 kg movies down a sloping path on a skate board. the sloping path makes an angle 45 degree with the horizontal. the constant speed of the child along the path is 6.5 m/s. Calculate:
a) vertical dist through which child moves in 1.0s (done)
b) the rate at which potential energy is being lost (g=9.81 m/s^2) (confusion)

How is the answer to b) 80 J/s??

All I did was P = (35x9.81x0.51)/1 which gives answer 175 J/s (or 175 W)